I Googled it. Nobody else has made this sequence up before. So it's mine. I'm naming it after me. You heard it here first.
I call it NAL's Double Cross Sequence, and it goes like follows:
5, 21, 45, 105, 405, 525, 945, 945, 2205... (as far as I've calculated).
And the description I've given this sequence is as follows:
For each term t[n], t[n] is the lowest number of squares that can be arranged into n different cross shapes with rotational symmetry of order 4.
Or in mathematical terms:
For each term t[n], t[n] is the lowest solution to n=w²+4(w*h), where w and h are positive integers, which can be expressed with n different pairs of w and h values.
Or in image terms:
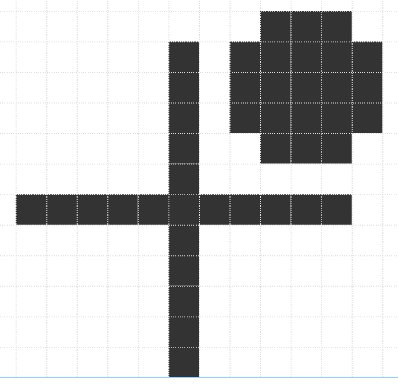
21crosses.png
That's a graphic representation of t[2], or 21. You can arrange 21 squares into two different cross (or plus) patterns which have branches of equal thickness and length. For the formula w²+4(w*h), the thin, large cross has a width of 1 and a height of 5 (counting only squares used beyond the central "square"), and for the stubby chubby one, a width of 3 and a length of 1.1²+4(1*5) = 1+4(5) = 1+20 = 213²+4(3*1) = 9+4(3) = 9+12 = 21
I've taken to calling 21 the first Double Cross number for that reason. 33 is the second one (1,8 and 3,2). The lowest even Double Cross number is 60 (2,7 and 6,1).
The first Triple Cross number is 45 (1,5; 3,3; 5,1) and the first Quadruple Cross number is 105 (1,26; 3,10; 5,6; 7,2). The first Cross number outright is 5, which is simply a width of 1 and a length of 1.
The one choice I made in defining the actual sequence was that the chosen number could contain MORE than n sets of widths and lengths. This is why 945 is both term 7 and 8 in the sequence - it can be written in 8 different ways, but there are no smaller numbers that can be written in exactly 7 different ways - 2025 is the smallest case.
What purpose does this serve? None that I can tell. Hooray for casual mathematics! But it's neat how the sequence works - that the differences between terms can fluctuate, even being 0 in some cases. And how it's (I would assume) totally possible for a term to be even, but they tend to be odd every time. Even the divisibility by 5, and even 105 - I'm not mathsy enough to explain it, but I like it.
If you want to play around with it further, here is a copy of the Excel spreadsheet I used to calculate the first nine terms of NAL's Double Cross Sequence. Sheet1 contains the raw values for pairs w,l, and Sheet2 contains the quantities of appearances of given numbers. You'll need to expand Sheet1 in both directions to get accurate readings in an expanded Sheet2, but it's all formulaed up!